How Quickly Do Bubbles Rise in a Pint of Beer?
The material we covered in the last two posts (skin friction and pressure drag) allows us to consider a fun little problem:
How quickly do the small bubbles of gas rise in a pint of beer?
To answer this question we will use the concept of aerodynamic drag introduced in the last two posts – namely,
- skin friction drag – frictional forces acting tangential to the flow that arise because of the inherent stickiness (viscosity) of the fluid.
- pressure drag – the difference between the fluid pressure upstream and downstream of the body, which typically occurs because of boundary layer separation and the induced turbulent wake behind the body.
The most important thing to remember is that both skin friction drag and profile drag are influenced by the shape of the boundary layer.
What is this boundary layer?
As a fluid flows over a body it sticks to the body’s external surface due to the inherent viscosity of the fluid, and therefore a thin region exists close to the surface where the velocity of the fluid increases from zero to the mainstream velocity. This thin region of the flow is known as the boundary layer and the velocity profile in this region is U-shaped as shown in the figure below.
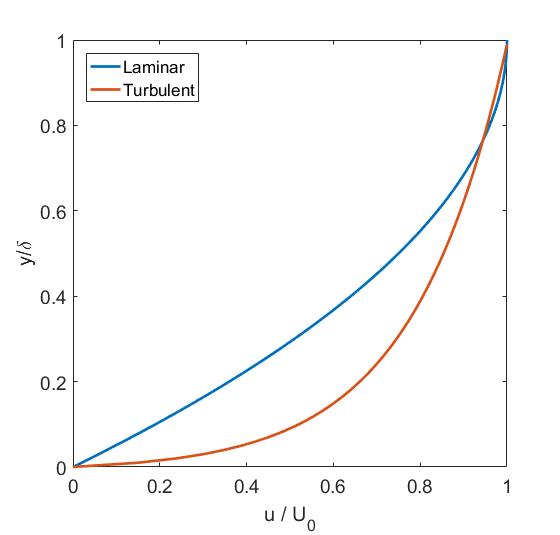
Velocity profile of laminar versus turbulent boundary layer
As shown in the figure above, the flow in the boundary layer can either be laminar, meaning it flows in stratified layers with no to very little mixing between the layers, or turbulent, meaning there is significant mixing of the flow perpendicular to the surface. Due to the higher degree of momentum transfer between fluid layers in a turbulent boundary layer, the velocity of the flow increases more quickly away from the surface than in a laminar boundary layer. The magnitude of skin friction drag at the surface of the body (y = 0 in the figure above) is given by
where is the so-called velocity gradient, or how quickly the fluid increases its velocity as we move away from the surface. As this velocity gradient at the surface (y = 0 in the figure above) is much steeper for turbulent flow, this type of flow leads to more skin friction drag than laminar flow does.
Skin friction drag is the dominant form of drag for objects whose surface area is aligned with the flow direction. Such shapes are called streamlined and include aircraft wings at cruise, fish and low-drag sports cars. For these streamlined bodies it is beneficial to maintain laminar flow over as much of the body as possible in order to minimise aerodynamic drag.
Conversely, pressure drag is the difference between the fluid pressure in front of (upstream) and behind (downstream) the moving body. Right at the tip of any moving body, the fluid comes to a standstill relative to the body (i.e. it sticks to the leading point) and as a result obtains its stagnation pressure.
The stagnation pressure is the pressure of a fluid at rest and, for thermodynamic reasons, this is the highest possible pressure the fluid can obtain under a set of pre-defined conditions. This is why from Bernoulli’s law we know that fluid pressure decreases/increases as the fluid accelerates/decelerates, respectively.
At the trailing edge of the body (i.e. immediately behind it) the pressure of the fluid is naturally lower than this stagnation pressure because the fluid is either flowing smoothly at some finite velocity, hence lower pressure, or is greatly disturbed by large-scale eddies. These large-scale eddies occur due to a phenomenon called boundary layer separation.
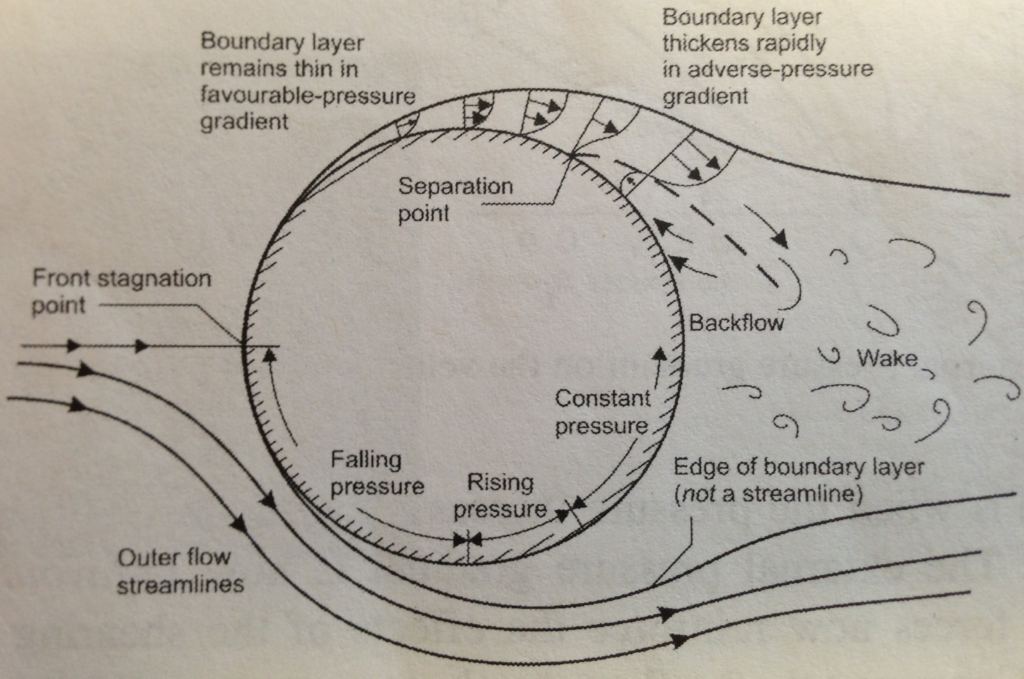
Boundary layer separation over a cylinder
Why does the boundary layer separate?
Any body of finite thickness will force the fluid to flow in curved streamlines around it. Towards the leading edge this causes the flow to speed up in order to balance the centripetal forces created by the curved streamlines. This creates a region of falling fluid pressure, also called a favourable pressure gradient. Further along the body, the streamlines straighten out and the opposite phenomenon occurs – the fluid flows into a region of rising pressure, also known as an adverse pressure gradient. This adverse pressure gradient decelerates the flow and causes the slowest parts of the boundary layer, i.e. those parts closest to the surface, to reverse direction. At this point, the boundary layer “separates” from the body and the combination of flow in two directions induces a wake of turbulent vortices; in essence a region of low-pressure fluid.
The reason why this is detrimental for drag is because we now have a lower pressure region behind the body than in front of it, and this pressure difference results in a force that pushes against the direction of travel. The magnitude of this drag force greatly depends on the location of the boundary layer separation point. The further upstream this point, the higher the pressure drag.
To minimise pressure drag it is beneficial to have a turbulent boundary layer. This is because the higher velocity gradient at the external surface of the body in a turbulent boundary layer means that the fluid has more momentum to “fight” the adverse pressure gradient. This extra momentum pushes the point of separation further downstream. Pressure drag is typically the dominant type of drag for bluff bodies, such as golf balls, whose surface area is predominantly perpendicular to the flow direction.
So to summarise: laminar flow minimises skin-friction drag, but turbulent flow minimises pressure drag.
Given this trade-off between skin friction drag and pressure drag, we are of course interested in the total amount of drag, known as the profile drag. The propensity of a specific shape in inducing profile drag is captured in the dimensionless drag coefficient
where is the total drag force acting on the body,
is the density of the fluid,
is the undisturbed mainstream velocity of the flow, and
represents a characteristic area of the body. For bluff bodies
is typically the frontal area of the body, whereas for aerofoils and hydrofoils
is the product of wing span and mean chord. For a flat plate aligned with the flow direction,
is the total surface area of both sides of the plate.
The denominator of the drag coefficient represents the dynamic pressure of the fluid () multiplied by the specific area (
) and is therefore equal to a force. As a result, the drag coefficient is the ratio of two forces, and because the units of the denominator and numerator cancel, we call this a dimensionless number that remains constant for two dynamically similar flows. This means
is independent of body size, and depends only on its shape. As discussed in the wind tunnel post, this mathematical property is why we can create smaller scaled versions of real aircraft and test them in a wind tunnel.
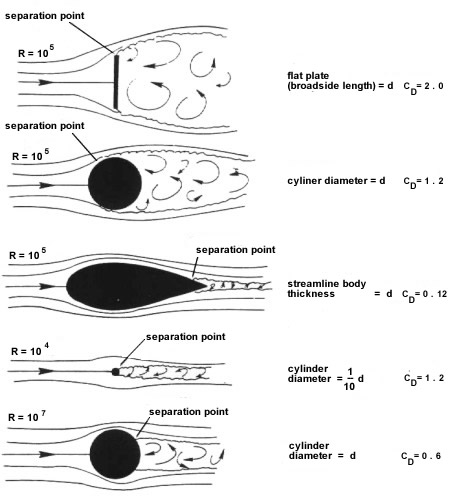
Looking at the diagram above we can start to develop an appreciation for the relative magnitude of pressure drag and skin friction drag for different bodies. The “worst” shape for boundary layer separation is a plate perpendicular to the flow as shown in the first diagram. In this case, drag is clearly dominated by pressure drag with negligible skin friction drag. The situation is similar for the cylinder shown in the second diagram, but in this case the overall profile drag is smaller due to the greater degree of streamlining.
The degree of boundary layer separation, and therefore the wake of eddies behind the cylinder, depends to a large extent on the surface roughness of the body and the Reynolds number of the flow. The Reynolds number is given by
where is the free-stream velocity and
is the characteristic dimension of the body. The reason why the Reynolds number influences boundary layer separation is because it is the dominant factor in influencing the nature, laminar or turbulent, of the boundary layer. The transition from laminar to turbulent boundary layer is different for different problems, but as a general rule of thumb a value of
can be used.
This influence of Reynolds number can be observed by comparing the second diagram to the bottom diagram. The flow over the cylinder in the bottom diagram has increased by a factor of 100 (), thereby increasing the extent of turbulent flow and delaying the onset of boundary layer separation (smaller wake). Hence, the drag coefficient of the bottom cylinder is half the drag coefficient of the cylinder in the second diagram (
) even though the diameter has remained unchanged. Remember though that only the drag coefficient has been halved, whereas the overall drag force will naturally be higher for
because the drag force is a function of
and the velocity
has increased by a factor of 100.
Notice also that the streamlined aircraft wing shown in the third diagram has a much lower drag coefficient. This is because the aircraft wing is essentially a “drawn-out” cylinder of the same “thickness” as the cylinder in the second diagram, but by streamlining (drawing out) its shape, boundary layer separation occurs much further downstream and the size of the wake is much reduced.
Terminal velocity of rising beer bubbles
The terminal velocity is the speed at which the forces accelerating a body equal those decelerating it. For example, the aerodynamic drag acting on a sky diver is proportional to the square of his/her falling velocity. This means that at some point the sky diver reaches a velocity at which the drag force equals the force of gravity, and the sky diver cannot accelerate any further. Hence, the terminal velocity represents the velocity at which the forces accelerating a body are equal to those decelerating it.

Turbulent wake behind a moving sphere. We will model the gas bubbles rising to the top of beer as a sphere moving through a liquid
The net accelerating force of a bubble of air/gas in a liquid is the buoyancy force, i.e. the difference in density between the liquid and the gas. This buoyancy force force is given by
where is the diameter of the spherical gas bubble,
is the density of the gas,
is the density of the liquid and
is the gravitational acceleration
. The buoyancy force essentially expresses the force required to displace a sphere volume
given a certain difference in density between the gas and liquid.
At terminal velocity the buoyancy force is balanced by the total drag acting on the gas bubble. Using the equation for the drag coefficient above we know that the total drag is
where is the terminal velocity and we have replaced
with the frontal area of the gas bubble
, i.e. the area of a circle. Thus, equating
and
and re-arranging for terminal velocity gives us
At this point we can calculate the terminal velocity of a spherical gas bubble driven by buoyancy forces for a certain drag coefficient. The problem now is that the drag coefficient of a sphere is not constant; it changes with the flow velocity. Fortunately, the drag coefficient of a sphere plateaus at around 0.5 for Reynolds numbers (see digram below) and it is reasonable to assume that the flow considered here falls within this range. Some good old engineering judgement at work!
Therefore, the terminal velocity of gas bubbles rising in a beer are somewhere in the range of
and taking the square root
Given that the viscosity of the fluid is around we can now check that we are in the right Reynolds number range:
which is right at the bottom of R = !
So there you have it: Beer bubbles rise at around a foot per second.
Perhaps the next time you gaze pensively into a glass of beer after a hard day’s work, this little fun-fact will give you something else to think (or smile) about.
Acknowledgements
This post is based on a fun little problem that Prof. Gary Lock set his undergraduate students at the University of Bath. Prof. Lock was probably the most entertaining and effective lecturer I had during my undergraduate studies and has influenced my own lecturing style. If I can only pass on a fraction of the passion for engineering and teaching that Prof. Lock instilled in me, I consider my job well done.
Related Posts
One Response to How Quickly Do Bubbles Rise in a Pint of Beer?
Leave a Reply Cancel reply
This site uses Akismet to reduce spam. Learn how your comment data is processed.
Sign-up to the monthly Aerospaced newsletter
Recent Posts
- Podcast Ep. #49 – 9T Labs is Producing High-Performance Composite Materials Through 3D Printing
- Podcast Ep. #48 – Engineering Complex Systems for Harsh Environments with First Mode
- Podcast Ep. #47 – Möbius Aero and MμZ Motion: a Winning Team for Electric Air Racing
- Podcast Ep. #46 – Tow-Steered Composite Materials with iCOMAT
- Podcast Ep. #45 – Industrialising Rocket Science with Rocket Factory Augsburg
Topics
- 3D Printing (4)
- Aerodynamics (29)
- Aerospace Engineering (11)
- Air-to-Air Refuelling (1)
- Aircraft (16)
- Autonomy (2)
- Bio-mimicry (9)
- Case Studies (15)
- Composite Materials (25)
- Composites (7)
- Computational Fluid Dynamics (2)
- Contra-Rotation (1)
- Design (2)
- Digitisation (2)
- Drones (1)
- Education (1)
- Electric Aviation (11)
- Engineering (23)
- General Aerospace (28)
- Gliders (1)
- Helicopters (3)
- History (26)
- Jet Engines (4)
- Machine Learning (4)
- Manufacturing (12)
- Military (2)
- Modelling (2)
- Nanomaterials (2)
- NASA (2)
- New Space (11)
- News (3)
- Nonlinear Structures (1)
- Novel Materials/Tailored Structures (14)
- Personal Aviation (5)
- Podcast (45)
- Propulsion (9)
- Renewable Energy (2)
- Renewables (1)
- Rocket Science (17)
- Satellites (8)
- Shape Adaptation (1)
- Smart Materials (1)
- Space (12)
- Space Junk (1)
- Sport Airplanes (2)
- Startup (19)
- STOL (1)
- Structural Efficiency (5)
- Structural Mechanics (1)
- Superalloys (1)
- Supersonic Flight (2)
- Technology (18)
- UAVs (2)
- Virtual Reality (2)
- VTOL (3)
- Privacy & Cookies: This site uses cookies. By continuing to use this website, you agree to their use.
To find out more, including how to control cookies, see here: Cookie Policy




I actually proposed this as my ME senior project at MSOE in 1988, after a night out drinking with classmates. Fortunately, or unfortunately, I was talked into examining the drag of spheres dropping in a column of liquid (not beer). It was a success, but not as interesting as studying bubbles in fluid or beer. I looked this study up after discussing my project with a coworker thirty two years later. I’ve only browsed the article, but will return for a deeper read. I like what I’ve read so far.